what is the circumference of a circle

When it comes to math, there are certain formulas and concepts that can be difficult to grasp, especially when you don’t have the right resources. That’s why we’re here to break down the concept of the circumference of a circle and provide you with easy-to-understand information and examples.
Circumference of a Circle (Perimeter of Circle) | Formula
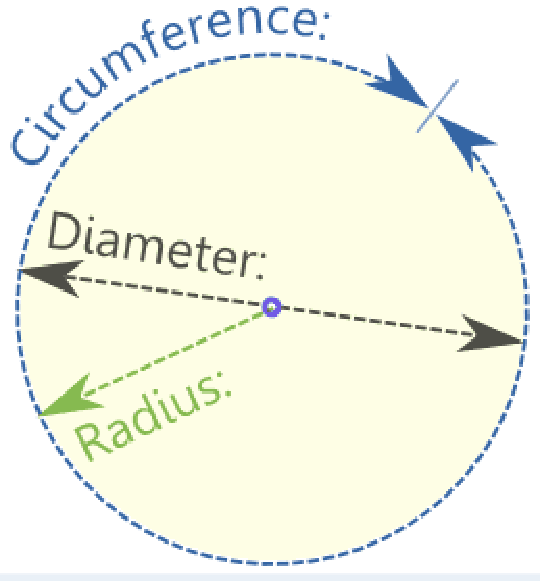
The circumference of a circle can simply be defined as the distance around the circle. It is the perimeter of the circle and can be calculated using the formula:
Circumference = 2πr or C = 2πr
Where π (pi) is a mathematical constant equal to approximately 3.14159 and r is the radius of the circle.

Abstract
In this article, we will be discussing the concept of the circumference of a circle and how to calculate it using its formula. We will also provide examples to help you better understand the concept.
Introduction
Circles are an important part of geometry and mathematics in general. They are used in various fields like architecture, engineering, physics, and even art. Understanding the different properties of a circle can help you in solving math problems and real-life scenarios.
One important property of a circle is its circumference, which is the distance around the circle. It is essentially the perimeter of the circle, just like how the perimeter of a square is the distance around it.
Content
To understand the concept of circumference better, let’s take a look at an example. Say we have a circle with a radius of 5 units. To find the circumference of the circle, we can use the formula given earlier:
C = 2πr
Plugging in the values we have, we get:
C = 2π(5) = 10π ≈ 31.41 units
Therefore, the circumference of the circle is approximately 31.41 units.
You may have noticed that we used the value of pi (π) in the formula. Pi is a mathematical constant that has a value of approximately 3.14159. It is an irrational number, which means it cannot be expressed as a simple fraction, and its decimal representation never repeats or ends.
The value of pi is crucial in not only finding the circumference of a circle but also its area, radius, and diameter. In fact, pi is used in many mathematical formulas and is an essential part of mathematics.
Now let’s take a look at another example. Say we have a circle with a diameter of 10 units. To find its circumference, we can use the following formula:
Circumference = πd or C = πd
Here, d represents the diameter of the circle. Plugging in the values we have, we get:
C = π(10) = 10π ≈ 31.41 units
As you can see, we get the same value for the circumference using both formulas. This is because the diameter is just twice the radius, so the same value of pi applies to both formulas.
It’s important to note that when working with circles, you should always use the same unit of measurement for both the radius/diameter and the circumference. For example, if the radius is measured in meters, then the circumference should also be measured in meters.
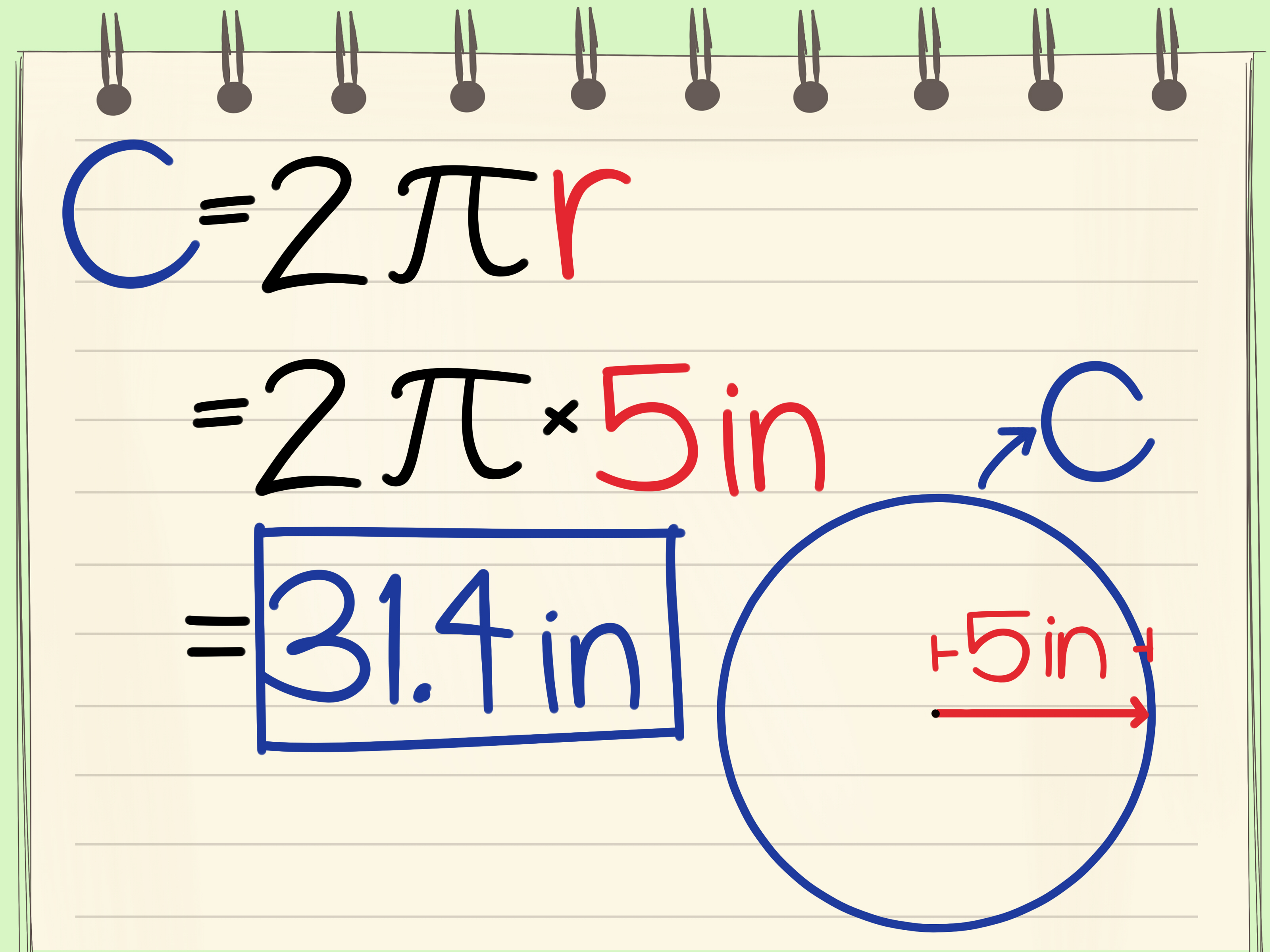
Now that we have a better understanding of the concept of circumference, let’s take a look at some real-life scenarios where it is used.
One common use of circles and their properties is in the design of circular objects like wheels, gears, and pulleys. The circumference of these objects needs to be carefully calculated to ensure a proper fit and function. For example, the circumference of a car wheel needs to match the diameter of the tire to ensure a smooth ride.
Another use of the circumference of a circle is in the calculation of the length of wire or rope needed to wrap around a circular object. This is useful in fields like construction and engineering where precise measurements are necessary.
The circumference of a circle can also be used in calculating the distance traveled around a circular path. For example, if you are running around a circular track, the distance traveled in one lap would be equal to the circumference of the track.
In addition to its practical uses, the concept of circumference is also important in mathematics and geometry. It is used in many mathematical formulas, like the formula for finding the area of a circle:
Area = πr²
Where r is the radius of the circle. The circumference is used in this formula to find the value of pi.
Conclusion
In conclusion, the circumference of a circle is an important concept in mathematics and has various real-life applications. It can be calculated using its formula, which involves the use of pi and the radius or diameter of the circle. Understanding this concept is essential in solving math problems and in fields like engineering, construction, and physics.
We hope this article has provided you with a better understanding of the concept of circumference. If you have any questions or would like to learn more about circles and their properties, feel free to do further research or consult with a math professional.

Source image : byjus.com
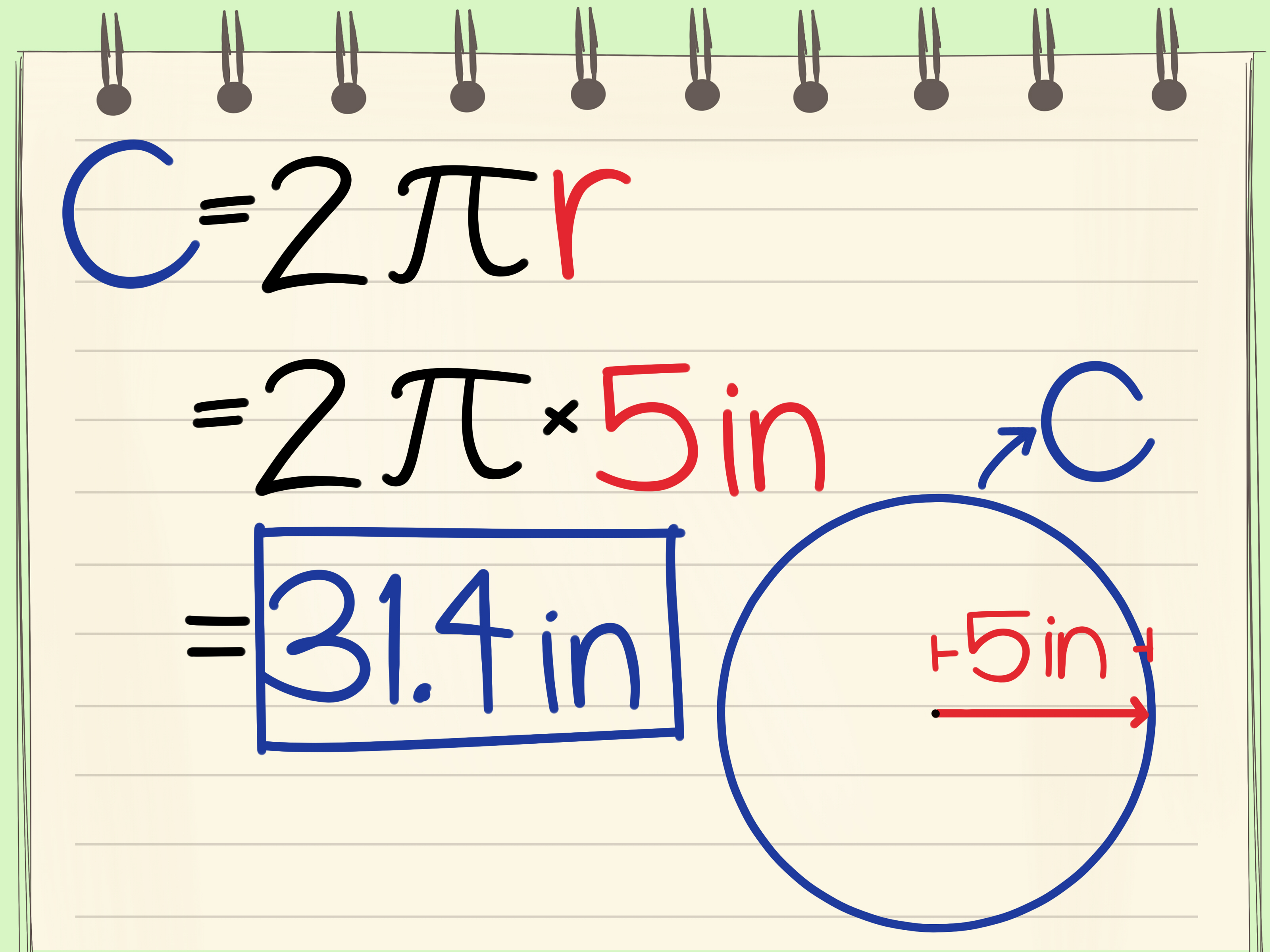
Source image : clipground.com
Source image : stickershock23.com






